Class9 Maths Ncert Exemplar with solution.ch13(Mensuration)
Hello students this is the full NCERT exemplar questions and answer with full details.
So you can download the pdf any time .
Class 9 Maths Ncert solution Exemplar will help you to give a overview of the chapter.
It will help a lot in the exams.
Perimeter of a closed figure is the distance covered in one round
along the boundary of the figure.
called a
• A closed figure in which all sides and angles are equal
regular polygon.
• Perimeter of a rectangle = 2 x (length + breadth)
• Perimeter of a square = 4x length of its side • Perimeter of an equilateral triangle = 3 x length of a side
The amount of region enclosed by a plane closed figure is called its area.
• Area of a rectangle = length x breadth
• Area of a square= side x side
(B) Solved Examples
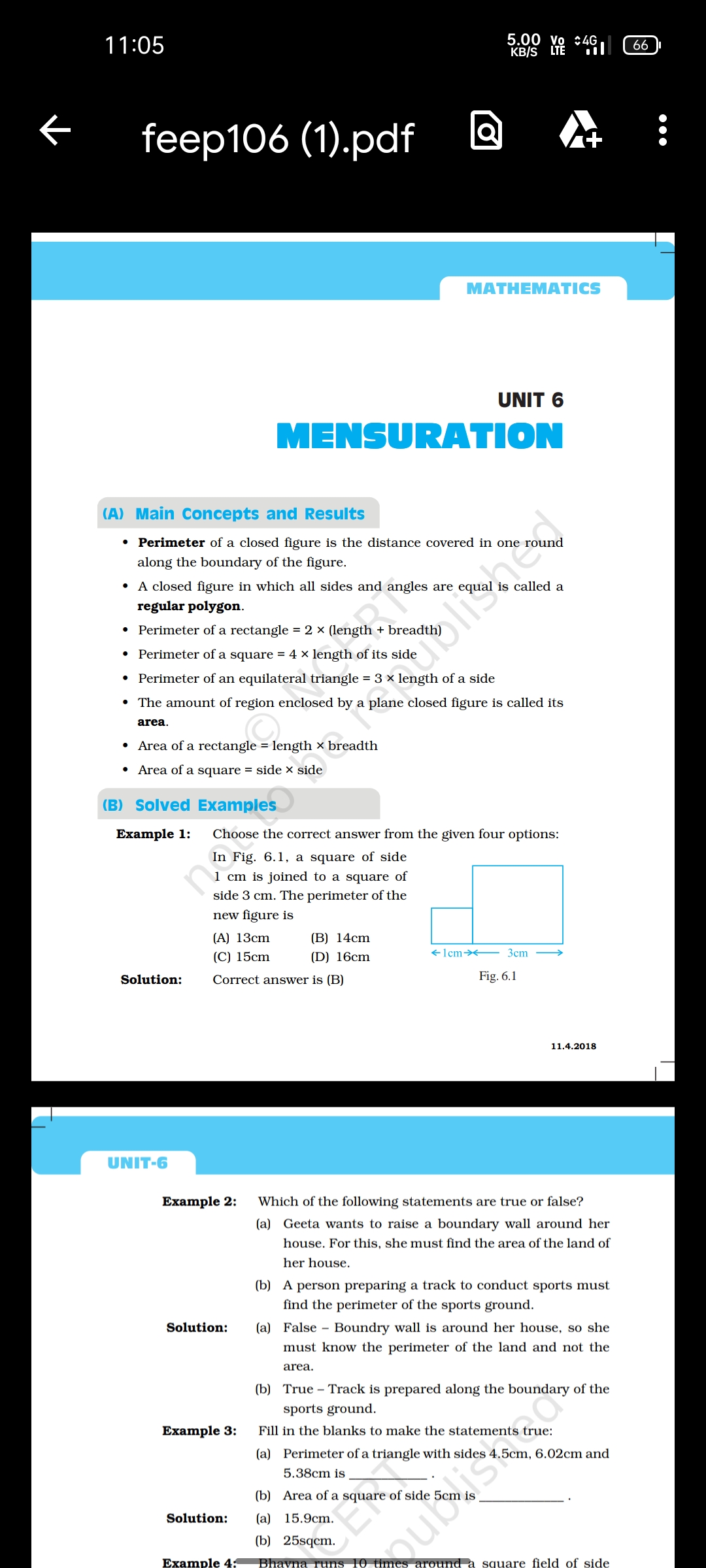
Example 1:
Choose the correct answer from the given four options:
In Fig. 6.1, a square of side
1 em is joined to a square of
side 3 cm. The perimeter of the
new figure is
(A) 13cm
(B) 14cm
(C) 15cm
(D) 16cm
Solution:
Correct answer is (B)
Fig. 6.1
11.4.2018
UNIT-6
Example 2:
Which of the following statements are true or false? (a) Geeta wants to raise a boundary wall around her
house. For this, she must find the area of the land of
her house.
(b) A person preparing a track to conduct sports must
find the perimeter of the sports ground. (a) False Boundry wall is around her house, so she must know the perimeter of the land and not the
Solution:
area. (b) True - Track is prepared along the boundary of the sports ground. છે.
Example 3:
Fill in the blanks to make the statements true:
(a) Perimeter of a triangle with sides 4.5cm, 6.02cm and 5.38cm is. OUીમાં
(b)
Area of a square of side 5cm
Solution:
(a) 15.9cm.
(b) 25sqcm.
Example 4:
Bhavna runs 10 times around a square field of side
80m. Her sister Sushmita runs 8 times around a rectangular field with length 150m and breadth 60m.
Who covers more distance? By how much? of the square field
Solution:
Distance covered by Bhavana in one round Perimeter
4 x side of square field = 4 x 80m = 320m
Distance covered in 10 rounds (320 x 10)m = 3200m not Distance covered by Sushmita in one round
= Perimeter of the rectangular field
= 2 x (length + breadth). = 2 x (150+60)m= 2 x 210 = 420m
Distance covered in 8 rounds 420 x 8 = 3360m
Distance covered in 8 rounds = 420 × 8 = 3360m
Hence, Sushmita has covered 160m more than the
distance coverd by Bhavna.
Example 5: The length of a rectangular field is thrice its breadth. If the eperimeter of this field is 800m, what is the length of the field?
Solution: Perimeter of a rectangle = 2 (length + breadth)
Length of the rectangular field = 3 × breadth
Therefore perimeter of field = 2 (3 × breadth +
breadth)
= 2 ( 4 × breadth)
= 8 × breadth
The given perimeter = 800m
Therefore 8 × breadth = 800
Or, breadth = 800 ÷ 8 = 100m
So, length = 3 × 100m = 300m
Example 6: Cost of fencing around a square field is Rs. 12000. If the cost of fencing per metre is Rs. 30, find the area of the square field.
Solution: Cost of fencing per metre = Rs 30
Total cost of fencing = Rs 12000
So, the length of fencing (perimeter) =
Total cost
Cost per metre
= = 400m
Now, length of fencing = Perimeter of the square field
= 4 × side of the field
Therefore, 4 × side of the field = 400m
or, side of the field = m = 100m
So, area of the field = 100m × 100m
= 10000sq m.
Example 7: Sabina wants to cover the floor of her room whose length is 4 m and breadth is 3m by square tiles. If each square tile is of side 20cm, then find the number of tiles required to cover the floor of her room.
Solution: Length of the room = 4m = 400cm
Breadth of the room = 3m = 300cm
Area of the floor of the room = Length × Breadth
400 × 300sqcm = 120000sqcm
Side of the square tile = 20cm
Area of the square tile = Side × Side = 20 × 20sqcm
= 400sqcm
So, number of tiles required = =
Example 8: By splitting the figure into rectangles, find its area.
Solution: By splitting the figure into four rectangles, we get
Fig. 6.3
Area of the figure = Area AJIY + Area YWCB
+ Area DWUE + Area FUHG.
Area A J I Y = A J × J I = 3 × 3 = 9
Now, B Y = A B – Y A = 4 – 3 = 1
So, Area Y W C B = B Y × B C = 1 × 2 = 2
Next, D W = D C + C W = 2 + 1 = 3
Therefore, area D W U E = D W × D E = 3 × 3 = 9
Similarly, U H = I H – I U = 4 – 2 = 2
G H = F U and F U = E U + F E
= D W + F E = 3 + 1= 4
Area F U H G = U H × G H = 2 × 4 = 8
Therefore, the area of the figure = 9 + 2 + 9 + 8
= 28sq unit
EXERCISE
In questions 1 to 6, out of the four options only one is correct. Write the correct answer.
1. Following figures are formed by joining six unit squares. Which figure
has the smallest perimeter in Fig. 6.4?
(i) (ii) (iii) (iv)
2)A square shaped park ABCD of side 100m has
two equal rectangular flower beds each of size
10m × 5m (Fig. 6.5). Length of the boundary of
the remaining park is
(A) 360m (B) 400m
(C) 340m (D) 460m
3. The side of a square is 10cm. How many times will the new perimeter
become if the side of the square is doubled?
(A) 2 times (B) 4 times (C) 6 times (D) 8 times
4. Length and breadth of a rectangular sheet of paper are 20cm and
10cm, respectively. A rectangular piece is cut from the sheet as shown
in Fig. 6.6. Which of the following statements is correct for the
remaining sheet?
10cm
20cm
5cm
2cm
Fig. 6.6
(A) Perimeter remains same but area changes.
Thanks for your valuable time.
You can check our MCQ sets.
Thanks.
By Sahil Patel



0 Comments